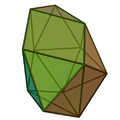
廣底加長型球狀屋頂 (日語:広底長球形屋根 、英語:Hebesphenomegacorona )是一種由18個三角形 和3個正方形組成的二十一面體[ 1] 詹森多面體 的其中一個,索引為J89 [ 2] 柏拉圖立體 (正多面體)和阿基米得立體 (半正多面體)經過切割、增補而得來,是詹森多面體中的基本立體之一。詹森多面體是凸多面體 ,面皆由正多邊形組成但不屬於均勻多面體,共有92種。這些立體最早在1966年由諾曼·詹森 [ 3]
廣底加長型球狀屋頂共由21個面 、33條邊 和14個頂點 所組成[ 4] [ 5] [ 6] [ 7] [ 5] [ 7] [ 7] 5 ]來表示[ 8] [ 7] 4 ,4]來表示[ 8] [ 7] 2 ,42 ]來表示[ 8]
若一個廣底加長型球狀屋頂邊長為
a
{\displaystyle a}
表面積
A
{\displaystyle A}
[ 9]
A
=
3
+
9
3
2
a
2
≈
10.7942
a
2
{\displaystyle A=3+{\frac {9{\sqrt {3}}}{2}}a^{2}\approx 10.7942a^{2}}
[ 10] 而其體積
V
{\displaystyle V}
a
3
{\displaystyle a^{3}}
[ 5]
令
a
{\displaystyle a}
[ 6]
26880
x
10
+
35328
x
9
−
25600
x
8
−
39680
x
7
+
6112
x
6
+
13696
x
5
+
2128
x
4
−
1808
x
3
−
1119
x
2
+
494
x
−
47
{\displaystyle {\begin{aligned}&26880x^{10}+35328x^{9}-25600x^{8}-39680x^{7}+6112x^{6}\\&\quad {}+13696x^{5}+2128x^{4}-1808x^{3}-1119x^{2}+494x-47\end{aligned}}}
則邊長為2的廣底加長型球狀屋頂的頂點座標為:[ 6]
(
±
1
,
±
1
,
2
b
)
{\displaystyle \left(\pm 1,\,\pm 1,\,2b\right)}
(
±
1
,
±
(
1
+
2
a
)
,
0
)
{\displaystyle \left(\pm 1,\,\pm \left(1+2a\right),\,0\right)}
(
±
(
1
+
c
1
−
a
)
,
0
,
−
2
a
2
+
a
−
1
b
)
{\displaystyle \left(\pm \left(1+{\frac {c}{\sqrt {1-a}}}\right),\,0,\,-{\frac {2a^{2}+a-1}{b}}\right)}
(
0
,
±
1
,
−
d
)
{\displaystyle \left(0,\,\pm 1,\,-d\right)}
(
±
d
c
+
e
f
e
,
0
,
(
2
a
−
1
)
d
f
−
c
f
e
)
{\displaystyle \left(\pm {\frac {dc+e}{fe}},\,0,\,{\frac {\left(2a-1\right)d}{f}}-{\frac {c}{fe}}\right)}
其中,
b
{\displaystyle b}
c
{\displaystyle c}
d
{\displaystyle d}
e
{\displaystyle e}
f
{\displaystyle f}
[ 6]
b
=
1
−
a
2
{\displaystyle b={\sqrt {1-a^{2}}}}
c
=
2
(
1
−
2
a
)
{\displaystyle c={\sqrt {2\left(1-2a\right)}}}
d
=
3
−
4
a
2
{\displaystyle d={\sqrt {3-4a^{2}}}}
e
=
1
+
a
{\displaystyle e={\sqrt {1+a}}}
f
=
2
(
1
−
a
)
{\displaystyle f=2\left(1-a\right)}
這些座標也可以由下列頂點的軌道 的並集在沿xz平面和yz平面鏡射所產生的空間對稱群 之群作用 下給出:[ 11]
(
1
,
1
,
2
1
−
a
2
)
,
(
1
+
2
a
,
1
,
0
)
,
(
0
,
1
+
2
2
a
−
1
a
−
1
,
−
2
a
2
+
a
−
1
1
−
a
2
)
,
(
1
,
0
,
−
3
−
4
a
2
)
,
(
0
,
2
(
3
−
4
a
2
)
(
1
−
2
a
)
+
1
+
a
2
(
1
−
a
)
1
+
a
,
(
2
a
−
1
)
3
−
4
a
2
2
(
1
−
a
)
−
2
(
1
−
2
a
)
2
(
1
−
a
)
1
+
a
)
{\displaystyle {\begin{aligned}&\left(1,1,2{\sqrt {1-a^{2}}}\right),\ \left(1+2a,1,0\right),\ \left(0,1+{\sqrt {2}}{\sqrt {\frac {2a-1}{a-1}}},-{\frac {2a^{2}+a-1}{\sqrt {1-a^{2}}}}\right),\ \left(1,0,-{\sqrt {3-4a^{2}}}\right),\\&\left(0,{\frac {{\sqrt {2(3-4a^{2})(1-2a)}}+{\sqrt {1+a}}}{2(1-a){\sqrt {1+a}}}},{\frac {(2a-1){\sqrt {3-4a^{2}}}}{2(1-a)}}-{\frac {\sqrt {2(1-2a)}}{2(1-a){\sqrt {1+a}}}}\right)\end{aligned}}}
廣底加長型球狀屋頂欠側錐(Diminished hebesphenomegacorona )是指從廣底加長型球狀屋頂上移除一個五角錐所構成的立體,然而,直接將五角錐從廣底加長型球狀屋頂移除將會出現一個不共面五邊形,無法構成多面體,需要將頂點位置些微調整,才能將五邊形面放置到移除五角錐的位置,這將導致廣底加長型球狀屋頂欠側錐的面僅是很接近正多邊形而不是正多邊形,因此是一種擬詹森多面體 。
廣底加長型球狀屋頂
廣底加長型球狀屋頂欠側錐
廣底加長型球狀屋頂欠側錐的3D模型
^ Santiago Alvarez. Polyhedra in (Inorganic) Chemistry (PDF) . Electronic Supplementary Information for Dalton Transactions. 2005 [2022-09-25 ] . (原始内容存档 (PDF) 于2022-01-21). ^ Weisstein, Eric W. (编). Hebesphenomegacorona . at MathWorld Wolfram Research, Inc. (英语) . ^ Johnson, Norman W. Canadian Journal of Mathematics 18 : 169–200, MR 0185507 Zbl 0132.14603 doi:10.4153/cjm-1966-021-8 ^ V.Bulatov. sphenomegacorona . [2022-09-11 ] . (原始内容存档 于2022-09-11). ^ 5.0 5.1 5.2 David I. McCooey. Johnson Solids: Hebesphenomegacorona . [2022-09-07 ] . ^ 6.0 6.1 6.2 6.3 The Hebesphenomegacorona . qfbox.info. [2022-09-11 ] . (原始内容存档 于2022-09-11). ^ 7.0 7.1 7.2 7.3 7.4 Hebesphenomegacorona . polyhedra.tessera.li. [2022-09-11 ] . (原始内容存档 于2022-09-11). ^ 8.0 8.1 8.2 Richard Klitzing. hebesphenomegacorona, hawmco . bendwavy.org. ^ Wolfram, Stephen . " Hebesphenomegacorona" Wolfram Alpha : Computational Knowledge Engine, Wolfram Research (英语) . ^ Wolfram Research, Inc. Wolfram|Alpha Knowledgebase. Champaign, IL. 2020. PolyhedronData[{"Johnson", 89}, "SurfaceArea"] ^ Timofeenko, A. V. The non-platonic and non-Archimedean noncomposite polyhedra. Journal of Mathematical Sciences. 2009-10-17, 162 (5): 710–729. ISSN 1072-3374 S2CID 120114341 doi:10.1007/s10958-009-9655-0