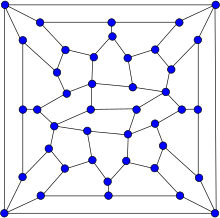
The Wiener–Araya graph is, in graph theory , a graph on 42 vertices with 67 edges. It is hypohamiltonian , which means that
it does not itself have a Hamiltonian cycle but every graph formed by removing a single vertex from it is Hamiltonian . It is also planar .
History
Hypohamiltonian graphs were first studied by Sousselier in Problèmes plaisants et délectables (1963).[ 1] [ 2] [ 3] [ 4]
From the start, the smallest hypohamiltonian graph is known: the Petersen graph . However, the hunt for the smallest planar hypohamiltonian graph continues. This question was first raised by Václav Chvátal in 1973.[ 5] Carsten Thomassen , who exhibited a 105-vertices construction, the 105-Thomassen graph .[ 6] planar hypohamiltonian graph on 57 vertices : the Hatzel graph .[ 7] 48-Zamfirescu graph .[ 8]
In 2009, a graph built by Gábor Wiener and Makoto Araya became (with its 42 vertices) the smallest planar hypohamiltonian graph known.[ 9] [ 10] 42 ) appears to be the
answer to The Ultimate Question of Life, the Universe, and Everything from The Hitchhiker's Guide to the Galaxy , a Douglas Adams novel. However, subsequently, smaller planar hypohamiltonian graphs have been discovered.[ 11]
References
^ Sousselier, R. (1963), Problème no. 29: Le cercle des irascibles , vol. 7, Rev. Franç. Rech. Opérationnelle, pp. 405– 406 ^ Lindgren, W. F. (1967), "An infinite class of hypohamiltonian graphs", American Mathematical Monthly 74 (9): 1087– 1089, doi :10.2307/2313617 , JSTOR 2313617 , MR 0224501 ^ Gaudin, T.; Herz, P.; Rossi (1964), "Solution du problème No. 29", Rev. Franç. Rech. Opérationnelle (in French), 8 : 214– 218 ^ Busacker, R. G.; Saaty, T. L. (1965), Finite Graphs and Networks ^ Chvátal, V. (1973), "Flip-flops in hypo-Hamiltonian graphs", Canadian Mathematical Bulletin 16 : 33– 41, doi :10.4153/cmb-1973-008-9 MR 0371722 ^ Thomassen, Carsten (1976), "Planar and infinite hypohamiltonian and hypotraceable graphs", Discrete Mathematics 14 (4): 377– 389, doi :10.1016/0012-365x(76)90071-6 , MR 0422086 ^ Hatzel, Wolfgang (1979), "Ein planarer hypohamiltonscher Graph mit 57 Knoten", Mathematische Annalen 243 (3): 213– 216, doi :10.1007/BF01424541 , MR 0548801 , S2CID 121794449 ^ Zamfirescu, Carol T.; Zamfirescu, Tudor I. (2007), "A planar hypohamiltonian graph with 48 vertices", Journal of Graph Theory 55 (4): 338– 342, doi :10.1002/jgt.20241 , MR 2336805 , S2CID 260477281 ^ Wiener, Gábor; Araya, Makoto (April 20, 2009), The ultimate question , arXiv :0904.3012 Bibcode :2009arXiv0904.3012W ^ Wiener, Gábor; Araya, Makoto (2011), "On planar hypohamiltonian graphs", Journal of Graph Theory 67 (1): 55– 68, doi :10.1002/jgt.20513 , MR 2809563 , S2CID 5340663 ^ Jooyandeh, Mohammadreza; McKay, Brendan D. ; Östergård, Patric R. J.; Pettersson, Ville H.; Zamfirescu, Carol T. (2017), "Planar hypohamiltonian graphs on 40 vertices", Journal of Graph Theory 84 (2): 121– 133, arXiv :1302.2698 doi :10.1002/jgt.22015 , MR 3601121 , S2CID 5535167
External links