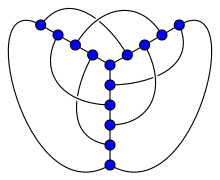
希伍德圖
cr
(
G
)
=
3
{\displaystyle {\text{cr}}(G)=3}
在圖論 ,交叉數
cr
(
G
)
{\displaystyle {\text{cr}}(G)}
圖
G
{\displaystyle G}
平面 上時,邊的交叉點的最小數目。若
cr
(
G
)
=
0
{\displaystyle {\hbox{cr}}(G)=0}
G
{\displaystyle G}
平面圖 。在圖形製圖 [ 1]
交叉數的研究始於圖蘭磚廠問題 圖蘭·帕爾 想求磚廠中,將每個窯爐各與全部貨倉用路軌連接的最優方案,使路軌的交叉儘可能少。按上述定義,即是問完全二部圖 的交叉數。[ 2] 社會學 研究提出,因為事關社會關係圖 [ 3] 完全圖 的交叉數公式亦然。
交叉數不等式 斷言,若邊數
e
{\displaystyle e}
n
{\displaystyle n}
e
3
/
n
2
{\displaystyle e^{3}/n^{2}}
超大规模集成电路 設計與組合幾何 方面有應用。
如無特別註明,交叉數允許使用任意曲線來畫邊。另一個相關概念是直線交叉數 ,其要求僅使用直線段來畫邊,所以未必等於交叉數。更具體說,完全圖
K
n
{\displaystyle K_{n}}
n
{\displaystyle n}
凸 四邊形 的最少數目,因為每個凸四邊形的兩條對角線產生一個交叉。直線交叉數問題與幸福結局問題 密切相關。[ 4]
給定一個圖,計算其交叉數是一個NP難 問題。[ 5]
定義交叉數之前,先定義無向圖 的畫法 。圖的畫法是一個映射,其將圖的頂點映到平面上互異的點,並將每條邊映到一條連接其兩端的曲線段,但頂點不能映到其他邊上(除非該邊以其為頂點),且若兩條邊的曲線段在公共頂點以外相交,則其僅產生有限多個交叉,並於每個交叉處橫截 [ 6]
一些作者對於畫法有更多限制,例如要求每對邊的交集至多只有一點(可為公共端點或交叉)。對於上段定義的交叉數,此項限制沒有任何影響,因為交叉最少的畫法當中,兩條邊必定相交至多一次。(若兩條邊有公共端點,而且相交,則可以將首個交點以前的兩段曲線互換,從而減少一個交叉。類似地,若兩條邊有兩個或更多交叉,則可以將相鄰兩個交叉之間的兩段曲線互換,以減少兩個交叉。)然而,若考慮交叉數的變式定義,例如只計算有多少對邊交叉(稱為兩兩交叉數 ),而非有多少個交叉,則上述限制確實會影響兩兩交叉數的值。[ 6]
截止2015年4月,僅得很少幾類圖的交叉數為人所知。即使是完全圖 、完全二部圖 、兩個環 的積等結構較簡單的圖,也僅得初始幾個的交叉數是已知的,但交叉數的下界方面,已有一定進展。[ 7]
K
4
,
7
{\displaystyle K_{4,7}}
在第二次世界大戰 期間,匈牙利數學家圖蘭·帕爾 被迫在磚廠工作,要將一車車的磚從窯爐推到貨倉。工廠的每個窯爐到每個貨倉之間各有一條路軌,而磚車在路軌交叉處特別難推。於是,圖蘭提出其磚廠問題 :窯爐、貨倉和路軌應如何安排,才能使交叉的數目最少?數學上,窯爐和貨倉可視為完全二部圖 的頂點,而路軌則是二部圖的邊。於是工廠的佈局就是該圖在平面上的一個畫法,換言之問題變成:
完全二部圖的畫法中,交叉的最少數目是多少?[ 8]
卡齊米日·扎蘭凱維奇 [ 9]
K
m
,
n
{\displaystyle K_{m,n}}
上界 :
cr
(
K
m
,
n
)
≤
⌊
n
2
⌋
⌊
n
−
1
2
⌋
⌊
m
2
⌋
⌊
m
−
1
2
⌋
.
{\displaystyle {\textrm {cr}}(K_{m,n})\leq \left\lfloor {\frac {n}{2}}\right\rfloor \left\lfloor {\frac {n-1}{2}}\right\rfloor \left\lfloor {\frac {m}{2}}\right\rfloor \left\lfloor {\frac {m-1}{2}}\right\rfloor .}
一個猜想是,上述上界確實是所有完全二部圖的交叉數。[ 10]
完全圖 的交叉數問題最先由安東尼·希爾 [ 11] 約翰·恩斯特 構成主義 藝術家。兩位不僅提出了問題,還猜想了該交叉數的公式,公式由理查·蓋 [ 11]
K
p
{\displaystyle K_{p}}
1
4
⌊
p
2
⌋
⌊
p
−
1
2
⌋
⌊
p
−
2
2
⌋
⌊
p
−
3
2
⌋
.
{\displaystyle {\frac {1}{4}}\left\lfloor {\frac {p}{2}}\right\rfloor \left\lfloor {\frac {p-1}{2}}\right\rfloor \left\lfloor {\frac {p-2}{2}}\right\rfloor \left\lfloor {\frac {p-3}{2}}\right\rfloor .}
上式在
p
=
5
,
6
,
⋯
,
12
{\displaystyle p=5,6,\cdots ,12}
1
,
3
,
9
,
18
,
36
,
60
,
100
,
150
{\displaystyle 1,3,9,18,36,60,100,150}
整數數列線上大全 的A000241 號數列。希爾等人猜想,不存在更好的畫法,即上式給出了完全圖的交叉數
cr
(
K
p
)
{\displaystyle {\text{cr}}(K_{p})}
湯瑪士·沙提 [ 12]
對於
p
≤
10
{\displaystyle p\leq 10}
p
=
11
,
12
{\displaystyle p=11,12}
[ 13]
2007年,米高·艾伯森(Michael O. Albertson)提出了艾伯森猜想 色數 為
p
{\displaystyle p}
K
p
{\displaystyle K_{p}}
p
{\displaystyle p}
p
≤
16
{\displaystyle p\leq 16}
[ 14]
已知交叉數為
1
{\displaystyle 1}
11
{\displaystyle 11}
立方圖 ,其頂點數分別為
6
,
10
,
14
,
16
,
18
,
20
,
22
,
24
,
26
,
28
,
28
{\displaystyle 6,10,14,16,18,20,22,24,26,28,28}
OEIS 數列A110507 ),如下表所記:
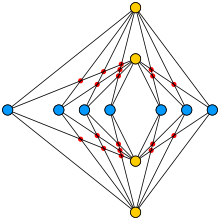
2009年,愛德華·柏奇 塔特-考克斯特圖 塔特12-籠 [ 16] [ 19]
一般情況下,很難計算圖的交叉數。米高·加里 大衛·詹森 NP難 問題。[ 5] 立方圖 [ 20] [ 21] 直線交叉數 (rectilinear crossing number)。該問題對於實數的存在理論 完全 的。[ 22]
另一方面,對於固定的常數
k
{\displaystyle k}
k
{\displaystyle k}
固定參數可馴順 [ 23] [ 24]
k
{\displaystyle k}
k
=
|
V
|
/
2
{\displaystyle k=|V|/2}
G
{\displaystyle G}
近似算法 能夠近似計算交叉數
cr
(
G
)
{\displaystyle {\text{cr}}(G)}
[ 25] 啟發式 算法,例如從空圖開始,逐條邊加入,使得每次產生的交叉數儘可能小。直線交叉數分布式计算 計劃(Rectilinear Crossing Number project)使用了此類算法。[ 26]
若無向 簡單圖
G
{\displaystyle G}
n
{\displaystyle n}
e
{\displaystyle e}
e
>
7
n
{\displaystyle e>7n}
交叉數
cr
(
G
)
{\displaystyle {\text{cr}}(G)}
不等式
cr
(
G
)
≥
e
3
29
n
2
.
{\displaystyle \operatorname {cr} (G)\geq {\frac {e^{3}}{29n^{2}}}.}
此種邊數、頂點數與交叉數的關係,最早由奧伊陶伊·米克洛什 瓦茨拉夫·赫瓦塔爾 蒙提·紐邦 塞邁雷迪·安德烈 四人[ 27] 湯姆森·雷頓 [ 28] 交叉數不等式 或交叉數引理。不等式的上述版本是為伊爾·艾克曼(Eyal Ackerman)的結果,其中常數
29
{\displaystyle 29}
[ 29]
7
{\displaystyle 7}
4
{\displaystyle 4}
29
{\displaystyle 29}
64
{\displaystyle 64}
[ 27] [ 28]
雷頓之所以研究交叉數,是為了理論計算機科學中,超大型積體電路 設計方面的應用。[ 28] 關聯幾何 方面,能夠簡短證明一些重要定理,例如塞邁雷迪-特羅特定理 和貝克定理 [ 30] 塔馬爾·戴伊 幾何k -集 [ 31]
若僅允許用直線段畫邊,而非任意曲線,則一些圖需要更多交叉才能畫出。對於此類畫法,交叉數目的最小可能值稱為直線交叉數 。直線交叉數必不小於交叉數,甚至對某些圖而言,直線交叉數嚴格大於交叉數。完全圖
K
5
{\displaystyle K_{5}}
K
12
{\displaystyle K_{12}}
1
,
3
,
9
,
19
,
36
,
62
,
102
,
153
{\displaystyle 1,3,9,19,36,62,102,153}
OEIS 數列A014540 )。已知直至
K
27
{\displaystyle K_{27}}
K
28
{\displaystyle K_{28}}
分布式计算 計劃(Rectilinear Crossing Number project)收集了更多數據。[ 26]
若一個圖有畫法使得每條邊至多
k
{\displaystyle k}
k
{\displaystyle k}
k
{\displaystyle k}
局部交叉數 (local crossing number)。若圖有畫法使每條邊至多
k
{\displaystyle k}
k
{\displaystyle k}
平面圖 (
k
{\displaystyle k}
[ 32]
其他變式包括兩兩相交數 (pairwise crossing number,即任何畫法中,有交叉的邊對數目的最小可能值)和奇相交數 (odd crossing number,即任何畫法中,交叉次數恰為奇數的邊對數目的最小可能值)。奇相交數不大於兩兩相交數,兩兩相交數也不大於相交數。然而,哈納尼-塔特定理 [ 6] Schaefer (2014 , 2018 ) 綜述了許多變式。[ 33] [ 34]
^ Purchase, Helen C.; Cohen, Robert F.; James, Murray I. Brandenburg, Franz-Josef , 编. Graph Drawing, Symposium on Graph Drawing, GD '95, Passau, Germany, September 20-22, 1995, Proceedings. Lecture Notes in Computer Science 1027 . Springer: 435–446. 1995. doi:10.1007/BFb0021827 ^ Turán, P. A Note of Welcome . Journal of Graph Theory . 1977, 1 : 7–9. doi:10.1002/jgt.3190010105 ^ Bronfenbrenner, Urie . The graphic presentation of sociometric data. Sociometry. 1944, 7 (3): 283–289. JSTOR 2785096 doi:10.2307/2785096 The arrangement of subjects on the diagram, while haphazard in part, is determined largely by trial and error with the aim of minimizing the number of intersecting lines. ^ Scheinerman, Edward R. ; Wilf, Herbert S. The rectilinear crossing number of a complete graph and Sylvester's "four point problem" of geometric probability . American Mathematical Monthly . 1994, 101 (10): 939–943. JSTOR 2975158 doi:10.2307/2975158 ^ 5.0 5.1 Garey, M. R. ; Johnson, D. S. Crossing number is NP-complete. SIAM Journal on Algebraic and Discrete Methods. 1983, 4 (3): 312–316. MR 0711340 doi:10.1137/0604033 ^ 6.0 6.1 6.2 Pach, J. ; Tóth, G. Proceedings of the 39th Annual Symposium on Foundations of Computer Science (FOCS 1998): 617–626. 1998. doi:10.1109/SFCS.1998.743512 ^ de Klerk, E.; Maharry, J.; Pasechnik, D. V.; Richter, B.; Salazar, G. Improved bounds for the crossing numbers of Km,n and Kn . SIAM Journal on Discrete Mathematics . 2006, 20 (1): 189–202 [2021-06-23 ] . S2CID 1509054 arXiv:math/0404142 doi:10.1137/S0895480104442741 存档 于2021-06-28). ^ Pach, János ; Sharir, Micha . 5.1 Crossings—the Brick Factory Problem. Combinatorial Geometry and Its Algorithmic Applications: The Alcalá Lectures. Mathematical Surveys and Monographs 152 . American Mathematical Society . 2009: 126–127. ^ Zarankiewicz, K. On a Problem of P. Turán Concerning Graphs. Fundamenta Mathematicae . 1954, 41 : 137–145. doi:10.4064/fm-41-1-137-145 ^ Guy, Richard K. The decline and fall of Zarankiewicz's theorem. Proof Techniques in Graph Theory (Proc. Second Ann Arbor Graph Theory Conf., Ann Arbor, Mich., 1968). Academic Press, New York. 1969: 63–69. MR 0253931 ^ 11.0 11.1 Guy, R. K. A combinatorial problem. Nabla (Bulletin of the Malayan Mathematical Society). 1960, 7 : 68–72. ^ Saaty, T.L. The minimum number of intersections in complete graphs . Proceedings of the National Academy of Sciences of the United States of America . 1964, 52 (3): 688–690. Bibcode:1964PNAS...52..688S PMC 300329 PMID 16591215 doi:10.1073/pnas.52.3.688 ^ Pan, Shengjun; Richter, R. Bruce. The crossing number of K 11 100 Journal of Graph Theory . 2007, 56 (2): 128–134. MR 2350621 doi:10.1002/jgt.20249 ^ Barát, János; Tóth, Géza. Towards the Albertson Conjecture. 2009. arXiv:0909.0413 math.CO ]. ^ Weisstein, Eric W. (编). Graph Crossing Number . at MathWorld Wolfram Research, Inc. (英语) . ^ 16.0 16.1 Pegg, E. T. ; Exoo, G. Crossing Number Graphs. Mathematica Journal. 2009, 11 (2). doi:10.3888/tmj.11.2-2 ^ 17.0 17.1 Sloane, N.J.A. (编). Sequence A110507 (Number of nodes in the smallest cubic graph with crossing number n.) . The On-Line Encyclopedia of Integer Sequences . OEIS Foundation. ^ Haythorpe, Michael; Newcombe, Alex, There are no Cubic Graphs on 26 Vertices with Crossing Number 11, 2018, arXiv:1804.10336 ^ Exoo, G. Rectilinear Drawings of Famous Graphs . [2021-06-24 ] . (原始内容存档 于2021-06-24). ^ Hliněný, P. Crossing number is hard for cubic graphs. Journal of Combinatorial Theory . Series B. 2006, 96 (4): 455–471. MR 2232384 doi:10.1016/j.jctb.2005.09.009 ^ Cabello S. and Mohar B. Adding One Edge to Planar Graphs Makes Crossing Number and 1-Planarity Hard. SIAM Journal on Computing . 2013, 42 (5): 1803–1829. S2CID 6535755 arXiv:1203.5944 doi:10.1137/120872310 ^ Schaefer, Marcus. Complexity of some geometric and topological problems (PDF) . Graph Drawing, 17th International Symposium, GS 2009, Chicago, IL, USA, September 2009, Revised Papers . Lecture Notes in Computer Science 5849 . Springer-Verlag: 334–344. 2010 [2020-11-04 ] . ISBN 978-3-642-11804-3doi:10.1007/978-3-642-11805-0_32 存档 (PDF) 于2021-06-26). ^ Grohe, M. Computing crossing numbers in quadratic time. Journal of Computer and System Sciences . 2005, 68 (2): 285–302. MR 2059096 arXiv:cs/0009010 doi:10.1016/j.jcss.2003.07.008 ^ Kawarabayashi, Ken-ichi ; Reed, Bruce . Computing crossing number in linear time. Proceedings of the 29th Annual ACM Symposium on Theory of Computing : 382–390. 2007. ISBN 978-1-59593-631-8doi:10.1145/1250790.1250848 ^ Even, Guy; Guha, Sudipto; Schieber, Baruch. Improved Approximations of Crossings in Graph Drawings and VLSI Layout Areas. SIAM Journal on Computing . 2003, 32 (1): 231–252. doi:10.1137/S0097539700373520 ^ 26.0 26.1 Oswin Aichholzer. Rectilinear Crossing Number project . (原始内容 存档于2012-12-30). Rectilinear Crossing Number (页面存档备份 ,存于互联网档案馆 ) on the Institute for Software Technology at Graz, University of Technology (2009).^ 27.0 27.1 Ajtai, M. ; Chvátal, V. ; Newborn, M.; Szemerédi, E. Crossing-free subgraphs. Theory and Practice of Combinatorics. North-Holland Mathematics Studies 60 : 9–12. 1982. MR 0806962 ^ 28.0 28.1 28.2 Leighton, T. Complexity Issues in VLSI. Foundations of Computing Series. Cambridge, MA: MIT Press. 1983. ^ Ackerman, Eyal. On topological graphs with at most four crossings per edge (PDF) . 2013. (原始内容 (PDF) 存档于2014-07-14). ^ Székely, L. A. Crossing numbers and hard Erdős problems in discrete geometry. Combinatorics, Probability and Computing . 1997, 6 (3): 353–358. MR 1464571 doi:10.1017/S0963548397002976 ^ Dey, T. K. Improved bounds for planar k -sets and related problems. Discrete and Computational Geometry . 1998, 19 (3): 373–382. MR 1608878 doi:10.1007/PL00009354 ^ Ringel, Gerhard . Ein Sechsfarbenproblem auf der Kugel. Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg. 1965, 29 (1–2): 107–117. MR 0187232 S2CID 123286264 doi:10.1007/BF02996313 (德语) . ^ Schaefer, Marcus. The graph crossing number and its variants: a survey . The Electronic Journal of Combinatorics . 2014. DS21 [2021-06-25 ] . (原始内容存档 于2021-06-29). ^ Schaefer, Marcus. Crossing Numbers of Graphs . CRC Press. 2018.