Pendule double

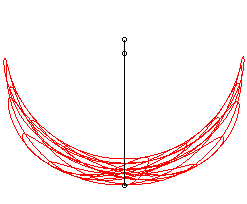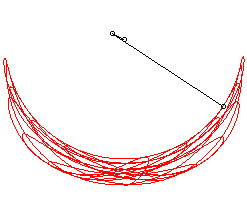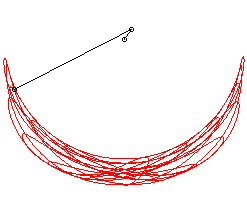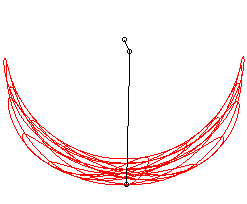
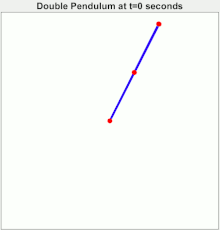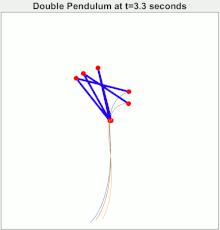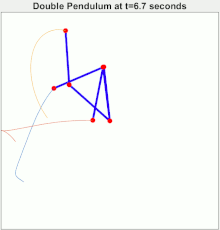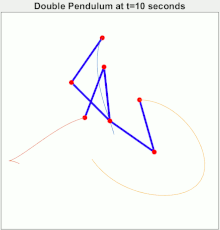
Illustration de la sensibilité aux conditions initiales avec trois pendules doubles aux conditions de départ très proches.

En mécanique, on désigne par pendule double un pendule à l'extrémité duquel on accroche un autre pendule. Son évolution est généralement chaotique.

Mise en équations

Un pendule double est un système dynamique typiquement limité à un mouvement plan. Il comporte deux degrés de libertés (${\displaystyle \theta _{1},\,\theta _{2}}$) et cinq paramètres (${\displaystyle l_{1},\,l_{2},\,m_{1},\,m_{2},\,g}$).

Approche lagrangienne

Le pendule est constitué de deux tiges de longueur ${\displaystyle l_{1}\,}$ et ${\displaystyle l_{2}\,}$, de masse nulle et deux masses ${\displaystyle m_{1}\,}$ et ${\displaystyle m_{2}\,}$.

L'énergie cinétique vaut :
${\displaystyle T={\frac {1}{2}}m_{1}v_{1}^{2}+{\frac {1}{2}}m_{2}v_{2}^{2}={\frac {1}{2}}m_{1}l_{1}^{2}{\dot {\theta }}_{1}^{2}+{\frac {1}{2}}m_{2}[l_{1}^{2}{\dot {\theta }}_{1}^{2}+l_{2}^{2}{\dot {\theta }}_{2}^{2}+2l_{1}l_{2}{\dot {\theta }}_{1}{\dot {\theta }}_{2}\cos(\theta _{1}-\theta _{2})]}$
où ${\displaystyle \theta _{i}\,}$ est l'angle par rapport à la verticale et ${\displaystyle v_{i}\,}$ la vitesse du pendule ${\displaystyle i\,}$.
L'énergie potentielle vaut :
${\displaystyle V=m_{1}gz_{1}+m_{2}gz_{2}\,}$ (${\displaystyle z_{i}\,}$ étant l'altitude de la masse ${\displaystyle i\,}$), ou
${\displaystyle V=-(m_{1}+m_{2})gl_{1}\cos(\theta _{1})-m_{2}gl_{2}\cos(\theta _{2})\,}$.
Le lagrangien vaut donc :
${\displaystyle L=T-V\,}$, soit
${\displaystyle L={\frac {1}{2}}(m_{1}+m_{2})l_{1}^{2}{\dot {\theta }}_{1}^{2}+{\frac {1}{2}}m_{2}l_{2}^{2}{\dot {\theta }}_{2}^{2}+m_{2}l_{1}l_{2}{\dot {\theta }}_{1}{\dot {\theta }}_{2}\cos(\theta _{1}-\theta _{2})+(m_{1}+m_{2})gl_{1}\cos(\theta _{1})+m_{2}gl_{2}\cos(\theta _{2})}$

En appliquant les équations de Lagrange, on obtient les équations du mouvement :

${\displaystyle {\begin{array}{l}(m_{1}+m_{2})l_{1}{\ddot {\theta }}_{1}+m_{2}l_{2}{\ddot {\theta }}_{2}\cos(\theta _{1}-\theta _{2})+m_{2}l_{2}{\dot {\theta }}_{2}^{2}\sin(\theta _{1}-\theta _{2})+(m_{1}+m_{2})g\sin(\theta _{1})=0\\l_{1}{\ddot {\theta }}_{1}\cos(\theta _{1}-\theta _{2})+l_{2}{\ddot {\theta }}_{2}-l_{1}{\dot {\theta }}_{1}^{2}\sin(\theta _{1}-\theta _{2})+g\sin(\theta _{2})=0\end{array}}}$

Dans le cas particulier ${\displaystyle m_{1}=m_{2},\,l_{1}=l_{2}}$, dans un système d'unités idoine, en renommant ${\displaystyle \theta _{1},\theta _{2}}$ en ${\displaystyle \phi ,\psi }$, et en ajoutant trois variables intermédiaires, ces équations deviennent :

${\displaystyle {\begin{array}{l}2{\ddot {\phi }}+c{\ddot {\psi }}+s{\dot {\psi }}^{2}+2\sin \left(\phi \right)=0\\{\ddot {\psi }}+c{\ddot {\phi }}+\sin \left(\psi \right)=s{\dot {\phi }}^{2}\\\delta =\phi -\psi \\c=\cos \left(\delta \right)\\s=\sin \left(\delta \right)\\\end{array}}}$

Aucune solution explicite n'étant connue, la résolution passe typiquement par l'utilisation de méthodes numériques.

Autour du minimum énergétique (voir section suivante), ce système possède des solutions périodiques décomposables en deux modes, mais il est chaotique, c’est-à-dire qu'il possède aussi des solutions ni périodiques ni pseudo-périodiques, mais présentant en permanence un mouvement original, et qu'il est alors sensible aux conditions initiales.

Approximation des petites oscillations

Le système est à l'équilibre pour ${\displaystyle \theta _{1}=\theta _{2}={\dot {\theta }}_{1}={\dot {\theta }}_{2}=0}$. Pour de petites oscillations autour de ces valeurs, nous pouvons introduire les approximations de MacLaurin ${\displaystyle \sin \theta =\theta }$ et ${\displaystyle \cos \theta =1}$. Les équations du mouvement peuvent alors être réduites au système linéaire:

${\displaystyle {\begin{alignedat}{5}(m_{1}+m_{2})l_{1}{\ddot {\theta }}_{1}&&\;+\;&&m_{2}l_{2}{\ddot {\theta }}_{2}&&\;+\;&&(m_{1}+m_{2})g\theta _{1}&&\;=\;&&0&\\l_{1}{\ddot {\theta }}_{1}&&\;+\;&&l_{2}{\ddot {\theta }}_{2}&&\;+\;&&g\theta _{2}&&\;=\;&&0&\end{alignedat}}}$

Le double pendule peut alors être analysé en termes de modes normaux, en remarquant que le système ci-dessus peut être réduit à la forme matricielle ${\displaystyle M{\ddot {\vec {\theta }}}+K{\vec {\theta }}={\vec {0}}}$.

Par exemple, pour ${\displaystyle m_{1}=m_{2}}$ et ${\displaystyle l_{1}=l_{2}=l}$, ce système s'écrit : ${\displaystyle {\begin{bmatrix}{\ddot {\theta }}_{1}\\{\ddot {\theta }}_{2}\end{bmatrix}}={\frac {g}{l}}{\begin{bmatrix}-2&1\\2&-2\end{bmatrix}}{\begin{bmatrix}\theta _{1}\\\theta _{2}\end{bmatrix}}}$

Approche Newtonienne

Les équations du mouvement peuvent également être trouvées en utilisant les complexes.

Représentons le double pendule ci-dessus dans le plan complexe de Gauss, en posant que l’axe des réels a même sens et même direction que la gravitation. Les points m₁ et m₂ représentant les mobiles 1 et 2 correspondent aux affixes z₁ et z₂. En fait, seuls les angles vont varier en fonction du temps puisque la masse et la longueur sont des constantes. Il faut donc chercher une manière de représenter les fonctions ${\displaystyle \theta _{1}}$ et ${\displaystyle \theta _{2}}$.

Dès lors, puisque le module de z₁ vaut l₁, son argument ${\displaystyle \theta _{1}}$, ${\displaystyle z_{1}=l_{1}(\cos \theta _{1}+i\sin \theta _{1})=l_{1}e^{i\theta _{1}}}$ . Ensuite, observons que z₂ est issu d’une translation de z₁ par le complexe z₀=${\displaystyle l_{2}e^{i\theta _{2}}}$ , c’est-à-dire un complexe tel que son module vaut l₂ et son argument ${\displaystyle \theta _{2}}$. En d’autres termes, z₂ = z₁ + z₀, donc ${\displaystyle z_{2}=l_{1}e^{i\theta _{1}}+l_{2}e^{i\theta _{2}}}$

Ici, les complexes z₁ et z₂ déterminent la position des mobiles1 et 2 en fonction du temps puisque ${\displaystyle \theta _{1}(t)}$ et ${\displaystyle \theta _{2}(t)}$ sont des fonctions du temps. L'accélération d'une masse mobile s'obtient en dérivant deux fois par rapport au temps la fonction définissant sa position. Ainsi, l’accélération de z₁ vaut

${\displaystyle a_{z_{1}}={\operatorname {d^{2}} \!z_{1} \over \operatorname {d} \!t^{2}}=l_{1}(i{\ddot {\theta }}_{1}-{\dot {\theta }}_{1}^{2})e^{i\theta _{1}}}$

et que celle de la deuxième est égale à

${\displaystyle a_{z_{2}}={\operatorname {d^{2}} \!z_{2} \over \operatorname {d} \!t^{2}}=l_{1}(i{\ddot {\theta }}_{1}-{\dot {\theta }}_{1}^{2})e^{i\theta _{1}}+l_{2}(i{\ddot {\theta }}_{2}-{\dot {\theta }}_{2}^{2})e^{i\theta _{2}}}$

Notons-les respectivement ${\displaystyle {\ddot {z}}_{1}}$et ${\displaystyle {\ddot {z}}_{2}}$.

Revenons dans la vie réelle. Quand une masse est suspendue à une corde, une tension se produit le long de celle-ci. Appelons, T₁ et T₂, les tensions exercées par les masses m₁ et m₂ et représentons-les sous forme de complexes t₁ et t₂.

Nous observons alors que t₁, z₁ et 0 sont alignés ou colinéaires, ce qui permet d’écrire que ${\displaystyle t_{1}=k_{1}z_{1}\,(k_{1}\in \mathbb {R} )}$. De même, t₂, z₀ et 0 sont alignés, ce qui permet d’affirmer que ${\displaystyle t_{2}=k_{2}z_{0}\,(k_{2}\in \mathbb {R} )}$.

La formule de la dynamique F = m a, connue aussi sous le nom de la 2^e loi de Newton, dit que la somme de toutes les forces appliquées à un mobile est égale au produit de l’accélération de celui-ci par sa masse.

Le mobile 2 est soumis à la tension T₂ et la force due à la gravité m₂ g, donnant les relations suivantes :

${\displaystyle F=ma_{z_{2}}=t_{2}+m_{2}g\Longleftrightarrow m_{2}{\ddot {z}}_{2}-m_{2}g=t_{2}\Longleftrightarrow {\ddot {z}}_{2}-g={\frac {t_{2}}{m_{2}}}={\frac {k_{2}z_{0}}{m_{2}}}\,\Rightarrow \,{\frac {{\ddot {z}}_{2}-g}{z_{0}}}={\frac {k_{2}}{m_{2}}}\,...(1)}$

Le mobile 1 est soumis à la tension T₁, à la force due à la gravité m₁g et à la tension T₂’ qui est issue du principe des actions réciproques (la 3^e loi de Newton) telle que T₂’= - T₂. Dès lors, nous pouvons dire que

${\displaystyle F=ma_{z_{1}}=t_{1}-t_{2}+m_{1}g\Longleftrightarrow t_{1}=t_{2}-m_{1}g+m_{1}{\ddot {z}}_{1}\Longleftrightarrow t_{1}=m_{2}{\ddot {z}}_{2}-m_{2}g-m_{1}g+m_{1}{\ddot {z}}_{1}}$

${\displaystyle \Longleftrightarrow m_{2}{\ddot {z}}_{2}-m_{2}g-m_{1}g+m_{1}{\ddot {z}}_{1}=k_{1}z_{1}\Longleftrightarrow {\frac {m_{2}{\ddot {z}}_{2}-m_{2}g-m_{1}g+m_{1}{\ddot {z}}_{1}}{z_{1}}}=k_{1}\,...(2)}$

Les membres de droite des équations (1) et (2) étant réels, exprimons que la partie imaginaire des membres de gauche est nulle :

Tout d’abord, concernant l’équation (1).

Calculons le membre de gauche.

${\displaystyle {\frac {{\ddot {z}}_{2}-g}{z_{0}}}={\frac {l_{1}(i{\ddot {\theta }}_{1}-{\dot {\theta }}_{1}^{2})e^{i\theta _{1}}+l_{2}(i{\ddot {\theta }}_{2}-{\dot {\theta }}_{2}^{2})e^{i\theta _{2}}-g}{l_{2}e^{i\theta _{2}}}}}$

${\displaystyle ={\frac {l_{1}i{\ddot {\theta }}_{1}\cos \theta _{1}-l_{1}{\dot {\theta }}_{1}^{2}\cos \theta _{1}-l_{1}{\ddot {\theta }}_{1}\sin \theta _{1}-l_{1}i{\dot {\theta }}_{1}^{2}\sin \theta _{1}+l_{2}i{\ddot {\theta }}_{2}\cos \theta _{2}-l_{2}{\dot {\theta }}_{2}^{2}\cos \theta _{2}-l_{2}{\ddot {\theta }}_{2}\sin \theta _{2}-l_{2}i{\dot {\theta }}_{2}^{2}\sin \theta _{2}-g}{l_{2}\cos \theta _{2}+l_{2}i\sin \theta _{2}}}}$

Appliquons le binôme conjugué pour supprimer le terme imaginaire au dénominateur, ce qui revient à multiplier la fraction par ${\displaystyle {\frac {\cos \theta _{2}-i\sin \theta _{2}}{\cos \theta _{2}-i\sin \theta _{2}}}}$.

Maintenant, seuls les imaginaires purs intéressent. C’est pourquoi, relevons uniquement les imaginaires purs issus du produit du numérateur par le conjugué du dénominateur. Nous conservons alors uniquement :

${\displaystyle (l_{1}i{\ddot {\theta }}_{1}\cos \theta _{1}-l_{1}i{\dot {\theta }}_{1}^{2}\sin \theta _{1}+l_{2}i{\ddot {\theta }}_{2}\cos \theta _{2}-l_{2}i{\dot {\theta }}_{2}^{2}\sin \theta _{2})(\cos \theta _{2})}$

${\displaystyle +(-l_{1}{\dot {\theta }}_{1}^{2}\cos \theta _{1}-l_{1}{\ddot {\theta }}_{1}\sin \theta _{1}-l_{2}{\dot {\theta }}_{2}^{2}\cos \theta _{2}-l_{2}{\ddot {\theta }}_{2}\sin \theta _{2}-g)(-i\sin \theta _{2})}$

Ainsi, nous pouvons affirmer que : 

${\displaystyle {\text{Im}}({\frac {{\ddot {z}}_{2}-g}{z_{0}}})}$

${\displaystyle ={\frac {l_{1}{\ddot {\theta }}_{1}\cos \theta _{1}\cos \theta _{2}-l_{1}{\dot {\theta }}_{1}^{2}\sin \theta _{1}\cos \theta _{2}+l_{2}{\ddot {\theta }}_{2}\cos ^{2}\theta _{2}-l_{2}{\dot {\theta }}_{2}^{2}\cos \theta _{2}\sin \theta _{2}}{l_{2}}}}$

${\displaystyle +{\frac {l_{1}{\dot {\theta }}_{1}^{2}\sin \theta _{2}\cos \theta _{1}+l_{1}{\ddot {\theta }}_{1}\sin \theta _{1}\sin \theta _{2}+l_{2}{\dot {\theta }}_{2}^{2}\sin \theta _{2}\cos \theta _{2}+l_{2}{\ddot {\theta }}_{2}\sin ^{2}\theta _{2}+g\sin \theta _{2}}{l_{2}}}}$

${\displaystyle ={\frac {l_{1}{\ddot {\theta }}_{1}\cos(\theta _{1}-\theta _{2})+l_{2}{\ddot {\theta }}_{2}-l_{1}{\dot {\theta }}_{1}^{2}\sin(\theta _{1}-\theta _{2})+g\sin(\theta _{2})}{l_{2}}}}$

Comme il faut que la partie imaginaire soit nulle, nous obtenons cette première équation différentielle.

  ${\displaystyle l_{1}{\ddot {\theta }}_{1}\cos(\theta _{1}-\theta _{2})+l_{2}{\ddot {\theta }}_{2}-l_{1}{\dot {\theta }}_{1}^{2}\sin(\theta _{1}-\theta _{2})+g\sin(\theta _{2})=0}$

Ensuite, concernant l’équation (2). Nous procédons de la même manière. Calculons le membre de gauche.

${\displaystyle {\frac {m_{2}{\ddot {z}}_{2}-m_{2}g-m_{1}g+m_{1}{\ddot {z}}_{1}}{z_{1}}}}$

${\displaystyle ={\frac {m_{2}[l_{1}(i{\ddot {\theta }}_{1}-{\dot {\theta }}_{1}^{2})(\cos \theta _{1}+i\sin \theta _{1})+l_{2}(i{\ddot {\theta }}_{2}-{\dot {\theta }}_{2}^{2})(\cos \theta _{2}+i\sin \theta _{2})]-m_{2}g-m_{1}g+m_{1}[l_{1}(i{\ddot {\theta }}_{1}-{\dot {\theta }}_{1}^{2})(\cos \theta _{1}+i\sin \theta _{1})]}{l_{1}\cos \theta _{1}+l_{1}i\sin \theta _{1}}}}$

${\displaystyle ={\frac {m_{1}[l_{1}i{\ddot {\theta }}_{1}\cos \theta _{1}-l_{1}{\dot {\theta }}_{1}^{2}\cos \theta _{1}-l_{1}{\ddot {\theta }}_{1}\sin \theta _{1}-l_{1}i{\dot {\theta }}_{1}^{2}\sin \theta _{1}]-m_{1}g-m_{2}g}{l_{1}\cos \theta _{1}+l_{1}i\sin \theta _{1}}}}$

${\displaystyle +{\frac {m_{2}[l_{1}i{\ddot {\theta }}_{1}\cos \theta _{1}-l_{1}{\dot {\theta }}_{1}^{2}\cos \theta _{1}-l_{1}{\ddot {\theta }}_{1}\sin \theta _{1}-l_{1}i{\dot {\theta }}_{1}^{2}\sin \theta _{1}+l_{2}i{\ddot {\theta }}_{2}\cos \theta _{2}-l_{2}{\dot {\theta }}_{2}^{2}\cos \theta _{2}-l_{2}{\ddot {\theta }}_{2}\sin \theta _{2}-l_{2}i{\dot {\theta }}_{2}^{2}\sin \theta _{2}]}{l_{1}\cos \theta _{1}+l_{1}i\sin \theta _{1}}}}$

Appliquons le binôme conjugué pour supprimer le terme imaginaire au dénominateur, ce qui revient à multiplier la fraction par${\displaystyle {\frac {\cos \theta _{1}-i\sin \theta _{1}}{\cos \theta _{1}-i\sin \theta _{1}}}}$.

Maintenant, seuls les imaginaires purs intéressent. C’est pourquoi, relevons uniquement les imaginaires purs issus du produit du numérateur par le conjugué du dénominateur. Nous gardons alors uniquement

${\displaystyle [m_{1}(l_{1}i{\ddot {\theta }}_{1}\cos \theta _{1}-l_{1}i{\dot {\theta }}_{1}^{2}\sin \theta _{1})+m_{2}(l_{1}i{\ddot {\theta }}_{1}\cos \theta _{1}-l_{1}i{\dot {\theta }}_{1}^{2}\sin \theta _{1}+l_{2}i{\ddot {\theta }}_{2}\cos \theta _{2}-l_{2}i{\dot {\theta }}_{2}^{2}\sin \theta _{2})][\cos \theta _{1}]}$

${\displaystyle +[m_{1}(-l_{1}{\dot {\theta }}_{1}^{2}\cos \theta _{1}-l_{1}{\ddot {\theta }}_{1}\sin \theta _{1})+m_{2}(-l_{1}{\dot {\theta }}_{1}^{2}\cos \theta _{1}-l_{1}{\ddot {\theta }}_{1}\sin \theta _{1}-l_{2}{\dot {\theta }}_{2}^{2}\cos \theta _{2}-l_{2}{\ddot {\theta }}_{2}\sin \theta _{2})-m_{1}g-m_{2}g][-i\sin \theta _{1}]}$

Ainsi, nous pouvons affirmer que

${\displaystyle {\text{Im}}({\frac {m_{2}{\ddot {z}}_{2}-m_{2}g-m_{1}g+m_{1}{\ddot {z}}_{1}}{z_{1}}})}$

${\displaystyle ={\frac {m_{1}l_{1}{\ddot {\theta }}_{1}\cos ^{2}\theta _{1}-m_{1}l_{1}{\dot {\theta }}_{1}^{2}\sin \theta _{1}\cos \theta _{1}+m_{2}l_{1}{\ddot {\theta }}_{1}\cos ^{2}\theta _{1}-m_{2}l_{1}{\dot {\theta }}_{1}^{2}\sin \theta _{1}\cos \theta _{1}+m_{2}l_{2}{\ddot {\theta }}_{2}\cos \theta _{1}\cos \theta _{2}}{l_{1}}}}$

${\displaystyle +{\frac {-m_{2}l_{2}{\dot {\theta }}_{2}^{2}\sin \theta _{2}\cos \theta _{1}+m_{1}l_{1}{\dot {\theta }}_{1}^{2}\sin \theta _{1}\cos \theta _{1}+m_{1}l_{1}{\ddot {\theta }}_{1}\sin ^{2}\theta _{1}+m_{2}l_{1}{\dot {\theta }}_{1}^{2}\sin \theta _{1}\cos \theta _{1}+m_{2}l_{1}{\ddot {\theta }}_{1}\sin ^{2}\theta _{1}}{l_{1}}}}$

${\displaystyle +{\frac {m_{2}l_{2}{\dot {\theta }}_{2}^{2}\sin \theta _{1}\cos \theta _{2}+m_{2}l_{2}{\ddot {\theta }}_{2}\sin \theta _{1}\sin \theta _{2}+m_{1}\sin \theta _{1}g+m_{2}\sin \theta _{1}g}{l_{1}}}}$

${\displaystyle ={\frac {(m_{1}+m_{2})l_{1}{\ddot {\theta }}_{1}+m_{2}l_{2}{\ddot {\theta }}_{2}\cos(\theta _{1}-\theta _{2})+m_{2}l_{2}{\dot {\theta }}_{2}^{2}\sin(\theta _{1}-\theta _{2})+(m_{1}+m_{2})g\sin(\theta _{1})}{l_{1}}}}$

Comme il faut que la partie imaginaire soit nulle, nous obtenons cette deuxième différentielle.

${\displaystyle (m_{1}+m_{2})l_{1}{\ddot {\theta }}_{1}+m_{2}l_{2}{\ddot {\theta }}_{2}\cos(\theta _{1}-\theta _{2})+m_{2}l_{2}{\dot {\theta }}_{2}^{2}\sin(\theta _{1}-\theta _{2})+(m_{1}+m_{2})g\sin(\theta _{1})=0}$

Nous avons donc pour finir :

${\displaystyle {\begin{cases}l_{1}{\ddot {\theta }}_{1}\cos(\theta _{1}-\theta _{2})+l_{2}{\ddot {\theta }}_{2}-l_{1}{\dot {\theta }}_{1}^{2}\sin(\theta _{1}-\theta _{2})+g\sin(\theta _{2})=0\\(m_{1}+m_{2})l_{1}{\ddot {\theta }}_{1}+m_{2}l_{2}{\ddot {\theta }}_{2}\cos(\theta _{1}-\theta _{2})+m_{2}l_{2}{\dot {\theta }}_{2}^{2}\sin(\theta _{1}-\theta _{2})+(m_{1}+m_{2})g\sin(\theta _{1})=0\end{cases}}}$

Ce sont les mêmes équations que pour l'approche lagrangienne.

Pendule à entraînement circulaire uniforme

Un autre exercice classique concerne le cas où la première tige se meut d'un mouvement uniforme autour de son axe. On a alors ${\displaystyle {\dot {\theta _{1}}}=\omega }$ et l'équation différentielle du mouvement, issue de (2), s'écrit, en posant ${\displaystyle \theta _{2}=\theta \,}$ :

${\displaystyle l_{2}{\ddot {\theta }}=-l_{1}\omega ^{2}\sin(\theta -\omega t)-g\sin \theta =-(1+{\frac {l_{1}\omega ^{2}}{g}}\cos \omega t)g\sin \theta +l_{1}\omega ^{2}\sin \omega t\cos \theta }$.

Pour de petites oscillations et ${\displaystyle {\frac {l_{1}\omega ^{2}}{g}}<<1\,}$, l'équation se linéarise en ${\displaystyle l_{2}{\ddot {\theta }}+g\theta =l_{1}\omega ^{2}\sin(\omega t)}$ et le système se comporte donc en oscillateur harmonique forcé :

Mais si, dans ce cas, on choisit ${\displaystyle \omega ={\sqrt {g/l_{2}}}\,}$, on obtient un phénomène de résonance ; par définition, les petites oscillations ne restent pas petites, et l'on tombe en fait dans un mouvement chaotique :

On constate que le pendule fait le tour si ${\displaystyle l_{2}/l_{1}<4,3\,}$

Notes et références

Voir aussi

• Pendule simple

Liens externes

• L'article de scienceworld d'Eric Weisstein, en anglais
• Manipulation interactive du pendule double
• Vidéo : mouvement chaotique du double pendule
• Animation flash du botafumeiro